[Full Table of Contents]
19. Continuity and Movement
19.1 Quantum Mechanical Objections to the Continuum
19.2 Infinite Divisibility of Spatial and Temporal Magnitude
19.3 Finitude of Rate of Movement
19.4 Indivisibility of the Present
19.5 Divisibility of the Mobile and the Changeable
19.6 Divisibility of Movement
19.7 Ends and Beginnings of Change
19.8 Late Medieval Considerations of First and Last Instants of Change
20. Modern Mathematics and Continuity
21. Coming to Rest
22. Finite and Infinite Distance and Time
Movement, by definition, is a continuous change between termini across a continuum of intermediate states. (Ch. 8) Until now, we have supposed that all measurable physical changes are movements, since extensive measure requires a continuum. What if in fact this is only an approximation? Perhaps, on a fine enough scale, there are only discrete increments of quality, quantity, or location. In that case, movement would not be a seamless growth, but a series of abrupt jumps from one state to the next.
If we allow, however, that there is some continuous magnitude, i.e., extension, then that magnitude cannot be composed of indivisibles, even if we suppose that physical objects can only occupy discrete states. For instance, if we allow that space is really something that admits of extensive measure, then no set of discrete spatial states could constitute that entire measure, unless each such state were supposed to have some extension or continuous measure; i.e., each unit is x meters long. Physicists sometimes speak of infinitesimals
as though they were indivisible extensive magnitudes, but this is an improper shorthand. All extensions are infinitely divisible, and the infinitesimal
interval dx really denotes a limiting procedure of taking ever smaller intervals (generally to compare against differences in f(x) over those intervals). The only true indivisibles are points, but we cannot arrive at an extensive magnitude or line by merely aggregating points, or we would never have a set of more than measure zero. If we were to arbitrarily confine spatial states to some countable set of points, we would not abolish the reality of the continuum, but would only have introduced some apparent discontinuity or acausality into physics.
Worse, such a discontinuity in spatial (or qualitative or quantitative) state would imply a similar discontinuity in time. For motion, as Aristotle argues, cannot be composed of moments any more than a line is composed of points. If this were so, an object would be at rest at each moment, and, its motion being composed of these static moments, it would be at rest throughout the motion. Motion would be composed of non-motion.
Mathematicians may object that the set of real numbers is an extension or continuum, and every real number is representable as a point, implying that the extension is an aggregation of points. In fact, however, only countably many real numbers are rational, algebraic, or even computable. For the rest, we cannot define their value or their ordering with respect to the rationals by any algorithm, making their representability as points dubious to say the least. Moreover, since there are uncountably many irrational, transcendental and non-computable numbers, they cannot be collected by mere aggregation, which presupposes countability. Rather, they are defined by properties of a field.
Although motion cannot be composed of moments, it perhaps might be constructed from them, as we construct a line from a set of points along it. Yet the line is always something more than a mere aggregation of points, or it could never have non-zero extension. Thus spatial motion, and movement more generally, requires categorical transcendence of static moments or states.
We have noted (Sec. 13.5) that quantum theory might have grainy
time (and therefore motion) if there are minimal intervals in which there is no change in state (i.e., in physically measurable properties). During such intervals, however, the time evolution of the wavefunction or operator (in the Schrödinger or Heisenberg pictures respectively) still occurs continuously, so you still have a continuous change in potentiality or propensity for actual observable change. One might counter that it is not meaningful to speak of potentiality at a time between grains
when actualization is impossible. Yet potentiality is always defined with reference to future actualization, so such a counter-argument would prove too much, for then it would never be meaningful to speak of potentiality.
In any case, referring to time as grainy or discrete, though linguistically possible, corresponds to nothing that will withstand scrutiny. If physical time were truly grainy, we would have to introduce some meta-time to account for transitions between grains. Without such a meta-time, no change could happen at all, since within each grain, time would be at a standstill, which is contrary to the very notion of time. Each grain would be a static universe, having nothing to do with the next. If we say the grains are adjacent or in direct contact with each other, then we have re-introduced the continuum, the only notion that makes direct contact intelligible.
In short, truly grainy time is impossible and indeed inconsistent with the notion of time as a medium of change, and physicists who contend otherwise are actually talking about the limits of spatiotemporal measurement resolution, not the abolition of the continuum that makes all physics possible. For one thing, without the continuum we should logically have to do without calculus! For another, the position-momentum commutation relation for free particles in quantum mechanics mathematically requires a continuum of possible position and momentum states.
Quantization of states for bounded particles does not abolish the reality of the continuum for the quantized variable, as should be clear from the fact that there are no absolutely forbidden values (i.e., forbidden to any particle whatsoever), but the quantization is peculiar to each particular circumstance, e.g., the initial location or wavelength of some particle.
Moreover, if space or spacetime did in fact consist of grains, we should then ask how these grains are packed. Spherical grains would have spaces between them, while grains of any other shape would have some peculiar orientation, introducing an absolute sense of direction and therefore a preferred set of coordinate axes or reference frame, contrary to relativity. Even spherical grains must be packed in a certain way, with lines of symmetry defining an absolute direction.
Further, on the assumption of spatial granularity, our account of something as simple as waving your arm in an arc has an absurd interpretation: countless particles making quantum leaps from one location to the next. Yet quantum mechanics does not have such apparent leaps occur unless there is an interaction or measurement of some sort. It is vastly unparsimonious to suppose a conveniently coordinated collection of innumerable jumps. If parsimony counts for anything in physics, we should accept the reality of the continuum.
The reality of the continuum does not contradict our denial that there can be an actual infinity in nature. The infinity of the continuum is in potentiality, i.e., in the possibility of dividing it ad infinitum. We may treat all the possibilities of the continuum (be it spatial position or any other continuous variable) as a transfinite collection of points.[1] Only a finite number of these possibilities can be made actual at any time. In this view, the continuum of space should be regarded as potentiality. The continuous line is composed
of the span of possibilities represented by points.
This analysis is consistent with a dispositional interpretation of spatial properties, especially in quantum mechanics, as proposed by Karl Popper and others.[2] Disposition or potentiality evolves continuously. When we recognize potentiality as a real disposition or propensity, something more than the quantitative probability of actuality, there is no difficulty in admitting that the potentiality may evolve or change over time even as actuality is unchanged. In quantum mechanical terms, the propensities defined by the components of the wavefunction may vary over time, even if there is no measurement interaction to realize an actual state.
If we define the continuous as that which is divisible into parts that are infinitely divisible, it follows necessarily that time is continuous. This definition of continuity is consistent with modern developments in mathematics, since Cantor’s analysis of the continuum does nothing to abolish its infinite divisibility. In fact the infinite divisibility criterion provides a weaker condition than the modern definition, for even the set of rational numbers is infinitely divisible. Time, like space, is infinitely divisible in potentia. This is not contradicted by the theoretical possibilities of grainy
space and time, insofar as these deal only with a discrete number of actualized spatiotemporal states, still allowing for a continuum of potentiality.
Aristotle gives a proof in Book Zeta—which we here amplify and convert into modern notation—that the physical reality of unequal speeds implies the physical reality that spatial and temporal magnitudes are continuous, i.e., infinitely divisible. Suppose we have two bodies A and B, with A moving at a faster speed. If both bodies start from the origin and move to the destination x1, the faster body A will arrive first, which is to say that it needs less time to traverse the same distance as the slower body B. Since the slower body B will be behind A when A arrives at x1 at some time t1, B will have traveled a shorter distance in the same amount of time.
Thus we have two premises:
I. A faster body A travels the same distance as a slower body B in less time than B.
II. A slower body B travels less distance than a faster body A in the same amount of time.
With these premises laid, the proof of the infinite divisibility of time follows.
The last two steps can be repeated indefinitely, so both spatial and temporal magnitudes are infinitely divisible, given the reality of two bodies traveling at unequal velocities. We may further show that the sequences of ti and xi both converge to zero, so that eventually there will be some real magnitude of t or x that is smaller than any arbitrarily small but finite fundamental unit we might choose to define.
From the above deductions, and the premise that x = vt, we derive:
x1 = vAt1
x2 = vBt1 = vAt2
x3 = vBt2 = vAt3
x4 = vBt3 = vAt4…
From this we find that each successive value of ti differs from the previous by a fixed ratio:
t2 = (vB/vA)t1
t3 = (vB/vA)t2
ti+1 = (vB/vA)ti
This sequence obviously converges to a limit of zero, since vB/vA < 1. By symmetry, we could similarly show the convergence of xi.
The above argument does not require us to specify that velocity may take on any real value or any rational value, only that unequal uniform velocities are possible. Indeed, without such a supposition, our entire notion of inertial mechanics and everything derived therefrom would fall apart.
In relativity, any velocity v of a massive object is expressible as ratio with respect to c, the speed of light in a vacuum, yet the value of v depends on arbitrary choice of reference frame. If all reference frames are equivalent, then v can take any real value 0 ≤ v < c. If there were any forbidden values for v in this range, we would have entire classes of forbidden reference frames, and only those with permitted values of v could be physically real, contrary to the principle of relativity. Given our ability to define reference frames arbitrarily, v can take on any value in that range, so v/c can have any value 0 ≤ v/c < 1.
Once it is conceded that a ratio of velocities may have any real value between 0 and 1, we can construct another argument for the continuity of space and time, using a proof by contradiction. Suppose that there exists some finite increment of space or a finite increment of time, so that at least one of the two following statements is true in the rest frame of some subluminal object:
I. The position x of any physical event can only take discrete values xi.
II. The time t of any physical event can only take discrete values ti.
From this it would follow that the velocity of anything traveling between two events must be of the form v = xj/tj, where j is one of the discrete permitted values i for each variable. Yet there are only countably many such ratios, contradicting the thesis that v may take on any real value up to c.
It is no answer to suppose that only one of the two premises is true, allowing either space or time to be continuous. For we could always make a change of reference frame in which the discreteness of spatial (or temporal) intervals is transferred to temporal (or spatial) intervals, leading to the same paradox. Suppose x has only discrete values and t has continuous variables. Then under the Lorentz transformation:
t' = γ(t - vx/c2)
t', being dependent on x, can only take on discrete values. So the same contradiction results with the continuous values of v.
Likewise, if only t is discrete and x is continuous, we can take the transformation:
x' = γ(x - vt)
which introduces discreteness into space and yields the same contradiction.
If both time and space are grainy, we can map all possible values of place and time into the integers, so that all possible values of speed are a subset of rational numbers. This too falls short of the relativistic requirement that v can take any real value up to c.
Suppose we replaced the ostensibly uniform velocity v with abrupt stops and starts between discrete spatiotemporal points. In this way, v would again be free to take on any real value. Yet there is no reason for anything, much less everything, to stop and start thus. Moreover, what would one stop and move with respect to? Such a notion would reintroduce absolute rest. Once again, granular spacetime in the sense of denying any reality to a spatiotemporal continuum cannot be reconciled with the principle of relativity.
Zeno’s paradoxes of the dichotomy and Achilles and the tortoise are answerable because they confuse infinite divisibility with infinite extension. Both space and time are infinitely divisible, so such division can be accomplished while keeping them commensurable with a finite ratio, i.e., speed. There is no need for infinite time to traverse a finite distance. Aristotle thinks it is also evident that infinite time is needed only if you need to traverse an infinite distance. This supposes we are considering space only as a linear measure. Naturally we could have cyclical motion in a finite space over infinite time, though even here we have a parametrically infinite spatial trajectory.
Notably, Aristotle does not allow infinitesimal or infinite velocity, since this requires an incommensurable ratio. The distance traveled or time elapsed would be undefinable. Thus passage over a finite space cannot take infinite time (with uniform velocity), nor can passage over an infinite space occur in a finite time (even if velocity is not uniform). In each part of time, some length has been traversed. The finite length cannot use up the whole infinite time (per above), so it must use up only a finite time, and so on for other parts. The sum of finitely many finite parts is finite, not infinite.
If I have infinite speed, how far do I travel in one second? Two seconds? If I have infinitesimal speed, how long does it take to travel two meters? One meter? These questions indicate how such speeds are undefinable, and lead to paradoxical results. If we may travel the same infinite
distance in one second or two seconds or a trillion years, we arrive at the same place at many different times, which characterizes rest rather than motion. If we may travel one meter or two meters or a trillion meters in the same infinite
amount of time, we can be in many places at once. Nor would it be defined which place we reached first. One would have to abandon any notion of succession, which is to deny time, which is to deny physical motion.
Nothing temporal can have infinite speed, but the aeviternal,
having bounded duration without internal succession (see Sec. 13.5), can have infinite speed
from the perspective of the temporal. This is why the medieval Scholastics saw no philosophical impossibility in saintly bilocation. In Minkowski geometry, c is effectively an aeviternal speed, finite from our perspective, though nonetheless collapsing time in a now
, so that all places on a light-speed trajectory are here
for the photon. This comports with the classical optics model of light as a ray.
After establishing that space and time are infinitely divisible, Aristotle holds that the present or now
is indivisible. This is consistent because he views the present not as a measurable part of time, but as a limit or boundary between the past and future, partaking of neither. Since every point in time is successively present,
it follows that time is constituted of such indivisible moments
or instants, though not as a whole is composed of parts.
Given that a moment is infinitesimal in duration, nothing can be moving during a moment.
If motion within a moment were allowed, at some finite velocity, then something at higher velocity could move in a smaller amount of time, and the moment would be divisible.
As strange as it may sound for the present to be infinitesimal, the alternative is more problematic. If the present were a moment of finite duration, we could subdivide the duration into successive parts, and should have to say that the past, future or both was included in the present.
Strangely, the present, being an infinitesimal limit, spans no time. We only have time between two moments or nows.
This is another case of the problem of the continuum. Per Aristotle, the continuum is not composed of discrete quantities, but is infinitely divisible. Yet the spatial points or temporal moments are not parts that compose the continuum. Taken individually, they do not span any extension at all. To be precise, the now
is not really a point, but a limit or boundary between past and future. So past and future are in immediate succession, with the present being the boundary. He even says that the boundary of the past is the same as the boundary of the future, to leave no doubt of his intention.
This means that time is not composed of nows
or presents. None of these presents can be in immediate succession, just as no two points in the continuum can touch each other. Indivisibles have no borders to share. Time, if anything, is constituted of past and future intervals, connected continuously, the border between past and future being the present.
To be mobile (an object of local motion) implies existence in space, since place is the measure of the mobile qua mobile. To be able to move in the continuum of space, an object must be part of the continuum (i.e., have extension), and therefore divisible. It cannot be an infinitesimal point, line or surface.[3]
The indivisible (i.e., a line, point or surface considered in its infinitesimal dimension) is moved only accidentally, i.e., as limits or boundaries of extensions, or as points constituting a locus internus. ‘Indivisible’ may also refer to spiritual beings, lacking spatiality altogether. Here we make no judgment as to whether spiritual beings exist or what their nature would be, other than that they are immaterial, so the categories of extension (quantity) and space do not apply to them.
While the divisibility of the mobile is evident enough in the case of local motion, Aristotle makes a stronger claim, arguing that everything that changes must be divisible.
This perplexed later commentators, for continuity seems to be inapplicable to substantial change, i.e., generation and destruction, for a thing either is or is not. Various solutions were offered for this perceived problem, but Aquinas finds them to be unnecessary, for Aristotle is not asserting the divisibility of change as such, but of the changeable object. When an object undergoes change, even substantial change, it may be partly in one and partly at the other terminus. This can be the case when altering from black to white, as it may occupy the intermediate gray. In cases where there is no intermediate, as when going from the privation of fire to the form of fire, the object may yet be partly under the form of fire, not inasmuch as it is fire, but according to something connected with it, i.e., according to the particular disposition for fire, which disposition it partly receives before it has the form of fire.
(Comm. on Physics, 800) Thus the substantial change is divisible, not according to itself, but according to a motion connected with it. That is to say, some local motion or alteration connected with substantial change, being divisible, makes the latter divisible in a sense (dispositionally).
The imperfect applicability of continuity to substantial change undoubtedly accounts for Aristotle’s ambivalence about whether to include it as a type of kinesis. He clearly does include substantial change as kinesis in Book Gamma (discussed in Part II), but later denies that it is a type of movement, regarding it more generically as a change or metabole (as discussed in Sec. 16.3 and here). We may synthesize this by regarding substantial change as a continuous movement not per se but by association with an underlying movement that continuously changes the object’s disposition to receive a new form.
While this solves the difficulty of applying Aristotle’s argument to substantial change, it will not work in general for qualitative change or alteration. An object need not become hotter by successive parts becoming hot, but rather many parts at once may become hotter by degrees, so geometric continuity is at least partly irrelevant, and we could in principle have saltational changes in qualitative states. This indeed appears to be the case in quantum mechanics, though the phenomenon is conceivable even classically. Aquinas admits (as will Aristotle later in the Physics) that continuity is primarily and per se and strictly found only in local motion, which alone can be continuous and regular.
Thus the proofs given here apply to other movements not totally, but to the extent that they participate in continuity and regularity.
(Comm. on Physics, 802) This is not circular reasoning, for Aristotle is proving the continuity of the mobile by assuming the continuity of movement. This continuity is always true for local motion, by virtue of the continuity of space and time, but only sometimes true for qualitative alteration, so not all alterations are movements per se. For substantial change, it is true only insofar as this is mediated by local motion. Quantitative increase or decrease in spatial extension is always so mediated, so it is always continuous, but not all physical quantities are continuous-valued, so not all changes in quantity are movements.
Modern physicists may object to the inference that the continuity of space and time implies the continuity of local motion, however much this may seem to follow inexorably from abstract reasoning. Quantum mechanics, as commonly interpreted, seems to imply the impossibility of continuous trajectories. Strictly speaking, this need not be the case. At a minimum, quantum mechanics requires (assuming the principle of locality) that there can be no causally deterministic trajectory, i.e., one such that the position and momentum at each instant is strictly determined by prior values of position and momentum. We need not imply that a particle really leaps
in the sense of traversing a finite distance while never occupying any intermediate position. Alternatively, we could admit such leaps in the sense that a particle realizes a definite position (i.e., actualizes as a particle state) only upon interaction, and in between interactions (measurements) it only has the potential to realize itself in one of many possible positions, all of which, however, are limited by the finite velocity of light (or some lesser velocity depending on the particle type and energy).
Aristotle, of course, was not treating point-like particles, but objects with extensive magnitudes. In those cases, it is of course incontrovertible that mobile bodies are as divisible as the spatiotemporal continuum they inhabit. This does not mean that they cannot be composed of irreducible elements or atoms. Rather, it means that, by virtue of moving through continuous space and time, they must be capable of moving successively through the continuum in infinitesimal increments. To deny this would be to deny the reality of motion for macroscopic objects, and instead we would have to suppose that the entire object teleports saltationally, which is uncalled for even by quantum mechanics, as that would require an impossible degree of synchronization of quantum leaps among innumerable particles.
Movement (kinesis) as such, according to Aristotle, is divisible in two senses: (1) according to the time that it occupies, and (2) according to the several parts of the moving object.
The first sense, we may recall, is grounded in the notion that time is the measure of movement. Indeed, he considers the notions of before and after to pertain primarily to movements, and derivatively to time, which is the number of movement with respect to before and after. We might say mathematically that time applies an Archimedean ordering property to movements.
We have seen, however, that the duration of time between successive nows
is infinitely divisible, even though each now
or present is indivisible, being a limit rather than a duration. This is why Aristotle denies that any movement can occur in the now,
within which there is no before or after. An implication of this denial is that we cannot have a static account of reality (i.e., where only the present is real), for such an account would deny the reality of movement. We must instead consider reality to encompass the history of the cosmos and its contents.
If a movement spans the time from t1 to t2, during which the object moves from state A to state B, Aristotle considers that less than the full movement is completed in half the time, and even less of the movement in successively smaller intervals of time. Since time is infinitely divisible, so is movement. We cannot say for certain that movement and time are reduced in the same proportion, for the change from state A toward B could be more or less rapid in different time subintervals. We can say, however, that less than the full movement will be completed in a smaller time interval, and that with each reduction of the time interval from t1, an even lesser quantity of movement from A to B would be completed. We could avoid this conclusion only if we had the state remain unchanged during some finite duration, in which case it would properly be at rest, not in movement, for that subinterval.
Movement is divisible by parts of the thing moving. Here we consider movement as something quantifiable by the extension of the moving object, as in the Newtonian notion of momentum, or as in medieval impetus. The total movement of a body is the sum of the movements of its parts. Since a body is infinitely divisible, so too is its quantity of movement. As in the previous section, we note that this argument is only imperfectly applicable to movements other than local motions. For the local motion of bodies, it is applicable only insofar as it can be assumed that every part of a body has some mass.
In fact, mass is not distributed continuously across the volume of a body, though this may be a good macroscopic approximation. On subatomic scales, all mass is concentrated in point-like particles, which implies that the quantity of movement or momentum is only finitely divisible by extension. The minimum unit is not simply the momentum of a fundamental particle, for there are innumerable internal movements that effectively cancel each other out, and we are only concerned with those components that contribute to the momentum of the body as a whole. If a group of particles acts as a rigid body, we may consider the momentum to be continuously distributed across its entire volume, so that it is, at least for computational purposes, infinitely divisible. Yet this is a mere abstraction. The linear (or angular) momentum of a body is really the sum (including internal offsets) of the linear (or angular) momenta of its constituent particles.
Moreover, even when we consider a single fundamental particle, the angular momentum has a minimum quantity and can increase only in finite increments. The exact physical meaning of the intrinsic angular momentum or spin of a point-like particle is unclear, however, so characterizing it as a quantity of movement may be inapt, though it has dimensions of angular momentum, and this contributes to the angular momentum of a body (along with orbital angular momenta). More conservatively, we might regard the quantum spin as angular momentum only in an analogical sense, as being the equivalent amount of angular momentum needed to account for its magnetic moment, the degree to which it torques in a magnetic field.
Linear momentum is not quantized for free particles, but periodic structures may be explained in terms of a quantized linear momentum. Thus the linear momentum of a crystal lattice may be effectively quantized, not infinitely divisible.
Less controversially, Aristotle argues that the act of being moved is divisible to the same degree as the movement. So the act of being moved is infinitely divisible in the same sense as movement, that is, according to the infinite divisibility of time. Its divisibility according to parts of a body is subject to the same limitations mentioned for the divisibility of the quantity of movement as measured by momentum.
Aristotle’s notion that movement is divisible across space, abstracted from time, seems to be in tension with his denial that there can be any motion in a given instant. Modern calculus resolves this with its notion of instantaneous momentum in the differential form mdx/dt (classically). The instantaneous momentum is a sort of limit as the time interval becomes arbitrarily small, and is proportionate to the total motion of an object over some finite time.
By regarding movement as continuous, we necessarily situate it in some finite duration of time, rather than in an indivisible instant. When we examine the beginning or end of a movement, however, we should consider whether this initiation or termination ought to be considered instantaneous. Moreover, what is the state of the moved object with respect to the movement’s termini at the time movement is initiated and completed?
Aristotle approaches the problem by first establishing a thesis about change (metabole) in general. Every changing object O changes from some state (A) to some other state (B). By changing, it is departing from the terminus a quo (A), whether this departing is identical with changing or a consequence of it. Likewise, by having changed (i.e., having completed the change), it has departed from the terminus A, whether having departed
is identical with or merely consequent to having changed.
If the termini are contradictories, such that A is the privation of B, then O, by having changed,
i.e., having completed the change, has departed from A, which is to say it has departed from not being B, in which case it is B. Having proved this for a change from not-B (A) to B, it would also hold for a change from A to not-A (B), as having departed from A would imply that it is no longer A and is now not-A. If A and B are both positive entities, not mere privations of the other, but have no intermediary, the same logic holds, as the only alternative to being A is being B, and the object becomes B as soon as it is no longer A.
Let us consider the remaining kind of change, namely movement, where the termini A and B are distinct states, not necessarily contradictories, with a continuous interval of intermediate states. One may prove by contradiction that the object O must be in state B when the change has been completed. Suppose that the object O, upon having completed the change, should not be in B (the terminus ad quem), but in some other state that is neither A (from which it has departed) nor B; call it C. Since C is other than B and they are in a continuum, there must be some interval between C and B. Since B is the terminus ad quem, O must be changing toward it, yet this contradicts our supposition that the change has been completed. Thus for movement, as for all other kinds of change, the object must be in the terminus ad quem upon completion of the change, i.e., in the moment or instant immediately after the change is complete, since there is no change during an instant, for there is no duration within an instant. This instant is a limit or upper bound of the duration during which the change or movement occurs.
It remains to be proven that this first instant in which something has completed a change is truly indivisible. Aristotle calls this instant or moment the primary when
of the completion of the change. It is primary
in that it possesses this characteristic (containing the completion of the change) of itself and not by virtue of something pertaining to it. We may call it the most fundamental increment of time containing the completion of the change. If some time interval AC were divisible into AB and BC, and one of these subintervals contained the completion of the change, then AC certainly could not be the primary when
of the completion. Then we would have to see if either of these subintervals could be divided into smaller units, one of which contains the completion.
Any interval of finite duration AC, no matter how small, can be subdivided into two smaller intervals AB and BC. If the completion of change is in either of these intervals, then AC is not the primary when. If the change is ongoing throughout both subintervals (even if it ends at C), then this contradicts our implied assumption that AG contains only the completion of the change, for if it contained any other non-contemporaneous event, it would not be the primary when, for it contains the completion only by virtue of something pertaining to it (i.e., the endpoint C). Thus no divisible interval can be the primary when of the completion of a change, which leaves only an indivisible instant.
The above argument encompasses all changes except generation and destruction, but here it is all the more evident that a thing ceases to be at an indivisible instant and comes to be at an indivisible instant, for it is impossible that the same thing should both be (in the sense of actual existence) and not be in the same respect at the same time. Thus we need an indivisible instant to act as a limit between the time when something is X and not-X. This also assumes an excluded middle, or else we could have some interval where a thing is neither X or not-X. This is impossible if the ‘not’ signifies simple negation.
This argument does not abolish the possibility of generations or destructions effected by gradual, continuous processes. Sometimes we speak of the generation or destruction of what is actually a composite object, not corresponding to a single nature or physis. There need not be an indivisible instant where a tree ceases to be a tree, unless we have a rigid logical definition of such. A tree may lose its life and its form in some parts but not others, or in some processes but not others. What is fundamental is the natural movements within the tree, and the ceasing to be a tree is a macroscopic description of the effect. Nonetheless, it is invariably the case that a thing cannot actually be and not be X in the same respect at the same time.
No such impossibility applies to potential being. It is quite cogent for something to be in a state that has both the potential to realize X and the potential to realize not-X. This is the best way to account for so-called superpositions in quantum mechanics, instead of the clumsy description of being both alive and dead at the same time. A notion of being or existence that has no modal distinctions between actuality and potentiality is ill-suited to describe the realities indicated by quantum experiments.
We are unable, however, to identify a primary when
that contains the beginning of a process of change, i.e., a time in which the object is first changing. Although the end or completion of a change has a definite existence, and thus a definite moment of time in which it occurs, the beginning of a change has no existence: there is no such thing as the beginning of a process of change.
The reason for this lack of symmetry is that to begin to change is to be changing, and change requires some duration, which is infinitely divisible, whereas to complete a change is to cease changing, so there is no contradiction in there being some definite limit after the period of change.
To prove this by contradiction, let AD be the primary time
when a change begins. Thus in some preceding finite time interval CA the object O was at rest. If we supposed AD were an instant, such that A and D are simultaneous, we would have a contradiction, for O would be both at rest at A (since A is the end of the rest period CA) and it would have completed the change at A (which is simultaneous with D). This of course is a contradiction. Suppose that O were at rest in some state S during the time interval CA. Then it must be S at A. Yet if the change has also been completed at A, then it must also be in some other non-S state at time A, which is a contradiction. Perhaps we could avoid this conclusion by supposing that the instant A should not be included in the rest interval CA, in which case there is no final moment of rest. But this is just another way of saying there is no first moment of change.
If we instead allow that AD is some finite duration, then the object must be changing in every subinterval of AD. If there were any subinterval of AD where the object was not changing, then AD would not be the primary time of the beginning of change. Suppose AD is subdivided into AB and BD, but change starts to occur only within BD. Then BD becomes a candidate for the primary time of the beginning of change, yet we again run into the same conundrum, ad infinitum, due to the infinite divisibility of durations, unless at some point we arrive at some finite duration for which it could be said that the beginning
of change spans the entire duration. On such an assumption, however, change is occurring throughout this entire interval that is the putative primary time. Then we could subdivide this interval, and surely only the first segment would still contain the beginning of change, so infinite divisibility is not avoided.
The reason why Aristotle finds that the end of change is an indivisible limit, but the beginning of change is not, is that he reckons the beginning of change as being part of the process of change, therefore requiring duration, while the completion of change is its cessation, and therefore needs no duration.
Earlier, however, he had contended that rest, no less than movement, requires duration, for he reckons rest as something more specific than mere non-movement. Thus there is no contradiction with his regard of the completion or cessation of movement as instantaneous. Besides, there is no guarantee that the cessation of one movement is not immediately followed by some other movement rather than a period of rest.
There is a tension, nonetheless, between the claim that rest requires duration and the argument against the beginning of change being instantaneous, for this argument required us to suppose it was meaningful to speak of an object being at rest at some instant A. Aquinas indeed notices this:
It should be noted, however, that if a thing was at rest throughout an entire time, it does not follow that it was at rest in the last indivisible of that time; for we have already shown that in thenowthings are neither at rest nor in motion. But Aristotle concludes this here by arguing from what his adversary has proposed, namely, that the element of time in which the object was first being moved is an indivisible. And if it can be in motion in an indivisible of time, there is no reason why it could not also be at rest. (Comm. on Physics, 823)
This is a classical argumentum ad hominem, arguing according to the adversary’s assumption, namely that the primary time of the beginning of change AD may be collapsed into an instant A. If this is granted, there is no obstacle to allowing the preceding rest period CA to include the instant A. It has already been denied that A is a mere limit or boundary, leading to the contradiction of rest and movement abiding in the same moment.
Thus the time of movement should instead be regarded as an open interval (A, B), excluding the endpoints. The so-called end or completion of movement is at a definite time B, but the beginning of the movement has no definite time, since it is considered that to begin moving involves movement already.
Note that Aristotle does allow for instantaneous change, for not all changes are movements. In Book Theta, he will recommend the convention of regarding the instant of change as belonging to the new substance or quality that is the end of the change. This is consistent with his view that change primarily is said of the end and not the beginning.
Since rest requires duration, it likewise follows that there is no first instant in which rest begins. The time of rest excludes both endpoints of its duration, though the later endpoint may be considered the end of rest.
Walter Burley (1275-1344) considered that rest indeed could have a first and last instant of existence, for he defined rest as a mere privation of motion. Thus the absence of motion at its temporal endpoints sufficed to establish the presence of rest at those endpoints. Burley’s account might be problematic when there are successive movements, requiring us to posit an instant of rest between them.
The fact that there is no first instant of movement implies that there can be no primary part
of a body that changes first, in cases where change is by succession of parts, i.e., a body passing through some threshold. For if the entire body changes over some fixed time duration, then in half that duration, only a part of that body changes, and in ever smaller durations, ever smaller parts. Since the time of movement is infinitely divisible and so is movement, we will never arrive at a primary part
that changes absolutely first.
Again, this assumes the continuity of bodies. If in fact bodies are composed of fundamental particles that are point-like and indivisible, then there could be a first particle
that changes first. Since it is point-like, its change could be instantaneous. The continuity of change would just be an approximation based on treating a large group of particles as a continuous body.
The continuity of time and movement implies that anything that is moving necessarily has moved earlier. Consider some time interval AB to be the primary time
of a movement M, i.e., AB is the smallest time interval in which all of M occurs. Let us subdivide that interval at point C into AC and CB. If none of movement M occurs in either AC or CB, then AB would not be the primary time of M, contrary to our assumption. So M must occur in both AC and CB. Thus if the movement is occurring now at some point within CB, it certainly must already have moved some finite duration AC, during which the object completed some finite portion of the movement (or else it could not be said to have moved at all). We can in turn subdivide AC into two subintervals, and repeat the argument ad infinitum, so that no matter how close we are to the beginning of the movement, at any point in time when the movement is occurring, there will already have been some finite portion of movement that has already been completed. So there is no first
in movement as such. Moreover, the infinite divisibility of the continuum implies, according to Aristotle, that there would be infinitely many changes completed within a finite interval!
Against this we may push back on two fronts. First, we remark that Aristotle is only considering movements assumed to be continuous, which need not be the case for all actual physical processes. Aristotle himself acknowledges as much for generation and corruption, and for some qualitative changes. Here we are only considering changes in some continuous magnitude. The most unequivocal case would be local motion, for space itself is undoubtedly a continuum. Even here, however, quantum mechanics allows that the continuity of local motion is only in potential, not in actually realized trajectories, for localization of a field into a point-like particle requires some kind of interaction.
Second, we may find his interpretation of the continuum as containing infinitely many finite intervals to be problematic both mathematically and philosophically. In the latter aspect, it seems to run against the impossibility of the actual existence of the infinite, which Aristotle himself argued earlier in the Physics. Infinite divisibility is not problematic in this regard, insofar as the infinity of divisibility is merely potential, not actual. For any division of a finite interval, however, there will only be finitely many subintervals.
Again, Aquinas offers a solution by clarifying
Aristotle in a way that may actually correct him. He considers that a completed movement
is just a name for the terminus of that movement, so that the time of the completed movement is just a point (the terminus of the time interval represented as a line segment). Since there can be infinitely many such points within a segment, there can be infinitely many completed movements
so defined, which are really just limits by which time a given movement is definitely completed. Aquinas adds that this also shows how the argument may be applied to generation and corruption if they are regarded as including a prior continuous movement that ends in the being or non-being of something. If generation or corruption is considered merely as a terminus or limit in which something comes to be or not to be, then it is instantaneous.
The late Scholastics considered the problem of first and last instants as distinct for permanent,
successive,
and instantaneous
beings. Permanent beings are anything capable of remaining
for some duration, such as substances, certain qualities and other accidents. Successive entities are processes, such as movements, which are continuous changes over time. In this case, the problem is how to define a first or last instant of something whose essential being requires some duration. Lastly, most Scholastics followed Aristotle in affirming a class of entities that may exist only for an instant, and these instantaneous beings included temporal instants themselves. Notably, William of Ockham denied that temporal instants actually exist in the external world, considering them purely mental constructs.
Ockham also denied that movement is some thing in addition to the mobile object and its succession of states. Thus movement is not to be posited as some real successive being,
but is only a way of speaking about the mobile object and a succession of its states. The object and the states are the only real things. Yet the object and each state, considered in itself, is a permanent being, so we cannot arrive at process or successive being if we admit reality only to permanent beings. Ockham thinks he is giving a true exposition of Aristotle, and while these philosophers agree that the object and states are primary beings from which we infer the reality of movement, it does not follow that movement is not a distinct mode of being that adds to reality. Movement cannot be described in terms of any countable number of static states, but we need the addition of a continuity whereby they grow out of each other in time. Process or successive being is distinct from permanent and instantaneous beings, so the reality of movement requires us to posit an additional mode of being, if we are to escape the traps laid by Parmenides and Zeno.
Aristotle treats the first and last instants of permanent beings, especially the objects of movement or change, more fully in Book Theta, so we will defer our discussion of that problem. For now we simply note that Aristotle considered that the instant of change from one form or entity to another should belong to the later form or entity. Thus, insofar as the change between permanent beings is instantaneous, permanent beings have a first instant but no last instant. This opinion was followed by the late Scholastics William of Sherwood and Walter Burley, among others. Peter of Spain, by contrast, held that permanent things have both a first and last instant of being.[4]
The most influential late Scholastic work on first and last instants is Walter Burley’s lucid treatise De primo et ultimo instanti (c. 1315).[5] While dealing with the problem of instantaneous being and its corruption, Burley expounded a more general solution regarding the first and last instants of change and movement.
Aristotle assumes that instantaneous things do not go through a process of ceasing to exist, since they do not exist for any duration, so he goes immediately to the perfect tense when describing an instant that has ceased
to be. This raises the difficulties of what is meant by the corruption of an instant, if this is not a successive process, and whether this occupies any instant of time.
Burley addresses the problem by taking the now
as an exemplary instantaneous being. When has a particular now t ceased to be? If it ceases to be at t, i.e., when t is the present, this would make the now t both be and not be at the same instant t. If it ceases to be at some later time s, we again have a problem. Between any two instants t and s, there must be some other instant, in fact infinitely many. If the now
at t ceases to be at s, we must hold that it remains through all instants between t and s, which would make the now last longer than an instant. The now would then be a duration, not a limit. So it is impossible to identify a first instant when a particular now
has ceased to be.
Asserting that an instant is corruptible runs into the dilemma that the corruption (ceasing to be) occurs either when that instant is or when it is not (i.e., when it is past). Burley says the corruption occurs when the instant is. He says that the instant exists and is subject to corruption
at t, but he denies the implication that the instant has ceased to be.
Burley defines a notion of corruption for instantaneous things that does not involve process. He says an instantaneous thing ceases to be at the instant that it is, though it is only when it has ceased to be that it is not. Basically, an instant is not subject to corruption in the strict sense, but only in a broader sense. Corruption in the strict sense is a change (mutatio) from being to non-being. In a broad sense, it is a ceasing (desinitio) of the being of a thing, i.e., having non-being after being. This agrees with the notion that time itself cannot be the subject of change. Yet Burley goes further and says no instantaneous thing whatsoever is subject to corruption. (Aristotle says likewise in the Metaphysics.)
Corruption in the strict sense happens instantaneously; the change and having-been-changed (its terminus) are simultaneous. For this reason an instantaneous being cannot be subject to corruption in the strict sense. It would not be
in the only instant when it exists. A persistent being, by contrast, could exist some duration prior to its instant of corruption.
Burley explicitly says that being subject to corruption
in the broad sense means the same as ceasing to be.
How, then, do we avoid the conclusion that an instant ceasing to be
at the same time that it exists involves a contradiction? He says there are two senses of this ceases
: (1) this is and never afterwards will be; (2) this is not and this immediately beforehand was. Likewise, this begins
has two senses: (1) this is and never beforehand was; (2) this is not and this immediately afterwards will be. If we take both statements in their first sense, it is compatible to say both this instant begins
and this instant ceases
in the same instant, for both statements, taken in their first sense, imply that this instant is.
The choice of the first sense is not arbitrary in the case of instantaneous things, for only their non-being, not their being, can have extended duration, so only the first sense is applicable. The second sense of ceasing or beginning would require a thing to be or remain for some finite duration, however small. That sense may be applied to permanent beings (e.g., substances).
When has an instant first ceased to be? It is not possible for there to be a first instant where the instant t has been corrupted. We could always choose instants ever closer to t, yet slightly after t. Yet we can choose a first instant, namely t itself, where can truly say this instant ceases
in the first sense, i.e., this is and afterwards never will be. This passing from being to non-being does not occur through a change. Only the non-being of an instantaneous thing can have duration, so the time of non-being for an instant is an open set excluding its endpoint t. There is no first instant in this open set, thus no first instant in which t (or something existing only at t) has ceased to be.
Burley concurs with Aristotle’s claim in Book Zeta that successive entities, i.e., movements, have no first instant and no last instant (in the sense of primary time), because movement requires duration. With rest, however, he concludes oppositely, viz., rest has a first and last instant of existence. This is because he regards rest as a privation, and considers that privative things have beginnings and ceasing corresponding to the positive thing. Thus if the positive thing, namely movement, spans an open interval of time, with no first or last movement, then the time of rest immediately preceding or following that movement must include that interval’s endpoint. The alternative would be to admit an instant where there is neither rest nor movement, which is unacceptable given Burley’s definition of rest as a privation of movement. We have seen, by contrast, that rest is something more than the mere privation of movement, but entails remaining or permanence.
According to Burley, when one terminus of a movement is the non-existence of a quality, e.g., lowering in degrees of heat to zero, there can be no last instant of having some degree of heat, as there is no minimum (non-zero) quantity.[6]
From these considerations, Burley derives rules that summarize the principles of first and last instants for successive and permanent entities.
As noted previously, a new substance (the result of generation), has a first instant of being but not a last instant. A quality that varies by degrees may be interpreted as varying a form so that a being has successively different substantial forms. There can be a last instant of each determinate degree (assuming each degree is a specific quantity or quantitative range defining a form), and so of each determinate form, with the exception of a continuously varying degree that ends in zero.
While these definitions are certainly logically consistent, we cannot help but wonder if they are to some extent conventional and other equally valid conventions might have been chosen. We should inform this discussion with modern mathematical concepts of continuity and infinitesimals in order to ascertain whether and how first and last instants might be physically significant.
The problem of instants arises from treating time as an extensive measure, in combination with the Aristotelian claim that movement requires some finite duration. This raises two classes of problems: (1) in what sense, if any, is geometric extension composed of points or instants; (2) in what sense, if any, can change or movement be situated in an instant?
In Greek geometry, a part
(μερος) of a line, area, or volume must have some finite extension. Indeed, Euclid’s definition of a point is that which has no part.
If the intuition of a part is a share, and the measure of each share of a line is its length, then a point would be no share at all. Yet Euclid defines the ends of a line to be points. Although points are not part of a line, they are limits of a line. Analogously, a line or curve without breadth can act as a limit of some area, but it is not a part of the area. This accounts for Aristotle’s treatment of points or instants as limits rather than parts of spatial or temporal extension.
In Greek mathematics, extension and number are fundamentally different concepts. Lengths can be added to form longer lengths, but not multiplied except to form areas. Yet Descartes perceived that the proportionality of lengths in certain figures made possible the multiplication and division of lengths, here treating the values of lengths as numbers. Thus Descartes treated extensions not as such, but as representing numerical quantities, giving us the number line
that we take as intuitive,[7] as well as the Cartesian coordinate system. This representation may suggest that the line or extension is composed of numbers. Such a conception might work if each line segment represents a number, though in that case all such lengths (if measured from the same zero) would overlap. Descartes instead considered a number to correspond to the endpoint of each length, thus remaining with the classical conception of a point as a limit of some extension, rather than a component or part of extension.
Leibniz, inheriting the Cartesian representation of the x-y plane, invoked infinitesimals to represent the slope of the tangent to a curve. Two points A and B on that curve will have a difference in y coordinates and a difference in x coordinates. The slope of the line running through A and B will be the ratio of those differences. As A and B are chosen to be closer to each other, the computed slope may vary, ultimately approaching some limiting value as the distance from A to B approaches 0. This limiting value, when calculable, is the slope of the tangent at a point P (to which A and B converge), expressed as a ratio of infinitesimal differences dy/dx. If it seems strange that a ratio of infinitesimal differences can give a finite value, this is because dy and dx are not really definite values, but variable quantities. The difference in y and x coordinates between points along a curve may vary at different rates for each coordinate, and the slope expresses the ratio of these rates.
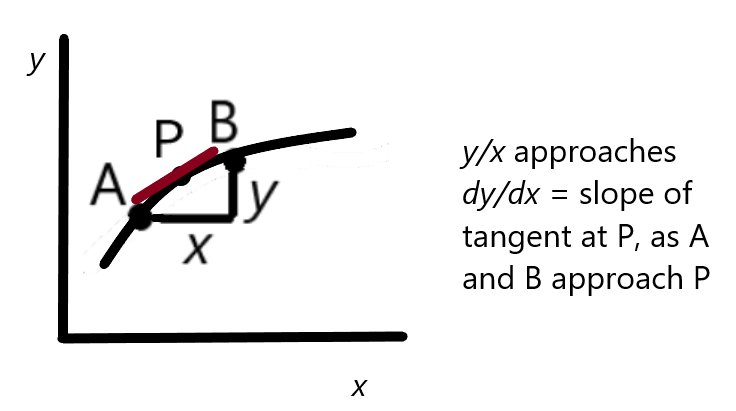
If we take y to represent an object’s position and x to represent time t, then the graph y(t) represents an object’s trajectory, and the slope dy/dt at some point on the curve gives the instantaneous velocity,
i.e., the velocity at some instant. This would seem to be contrary to Aristotle’s claim that there can be no movement in an instant.
Yet on closer inspection, the infinitesimals of Leibniz are not true infinitesimals or points. Unlike a Euclidean point, Leibniz’s infinitesimals are arbitrarily small intervals or differences. If the infinitesimal had no extension whatsoever, the difference dx would be zero, and dy/dx would be undefined. On the contrary, dy/dx can only have a definite value if x is allowed to vary, i.e., take different values, and then we take the differing values of y at those values of x (i.e., at our points A and B).
The tangent line at P is identifiable only by knowledge of the coordinate values at other nearby points A and B on the graph or trajectory. Thus instantaneous velocity is definable only by reference to some small interval or variation in the horizontal axis, representing time, so Aristotle’s claim that movement requires some temporal duration is unchanged.
Leibniz’s infinitesimals were also used to solve the problem of calculating the area under a curve, giving rise to integral calculus. The original interpretation of the Leibniz notation ∫ f(x) dx is that we are taking the sum of the product f(x) dx, where dx is an arbitrarily small horizontal interval of x and f(x) is the value (or y coordinate) of some function of x. The exact solution to ∫ f(x) dx is found by treating the area under the curve as approximated by these products and taking the limit as dx approaches zero. Again, dx cannot be identically zero, or we would have zero area.
Leonhard Euler (18th c.), finding that variables such as x, y and t represented quantities that may take different values, inferred that these variables must be subject to the same operations as fixed quantities. He treated infinitesimal quantities such as dx as equal to zero in the sense that c + dx = c for any finite constant c. As we have noted, however, treating dx as zero would be problematic in expressions such as dy/dx. Thus d’Alembert, by contrast, regarded infinitesimals not as quantities, but as limits of quantities, in the classical sense of limit as a boundary.
Augustin-Louis Cauchy (19th c.) discussed infinitesimals more rigorously in terms of a variable whose sequence of values tends towards zero, such that zero is the limit
of the sequence in the modern sense. The limit of a sequence need not be the value of any member of the sequence, as is evidently the case when the limit is infinite, for sequences increasing indefinitely. If zero is the limit of a sequence but not a member of the sequence, then we may say the sequence tends toward an infinitesimal quantity.
Cauchy’s notion of quantity
here embraces an unending process rather than a fixed value. An infinitesimal is a variable quantity, represented by the differing values of the sequence converging to zero.
David Tall and Mikhail Katz (2014) note that Cauchy’s notion of limit also had a geometric interpretation, as when a polygon approximates a circle as the number of sides increases. In this geometric view, the circle is the limit, a sort of barrier containing whatever finite-sided polygon approaches it. When speaking of numbers represented as points on a line, his infinitely small variable quantity
is a sequence of points successively closer to zero. Notably, Cauchy’s concept of limit, unlike the modern definition, did not require a unique value. Thus the function 1/x has two limits as x tends to zero, namely negative and positive infinity, depending on whether zero is approached from the left or the right. The limit of sin (1/x) has an infinity of values
between -1 and +1, oscillating ever more rapidly between these bounds as x tends to zero.
[8]
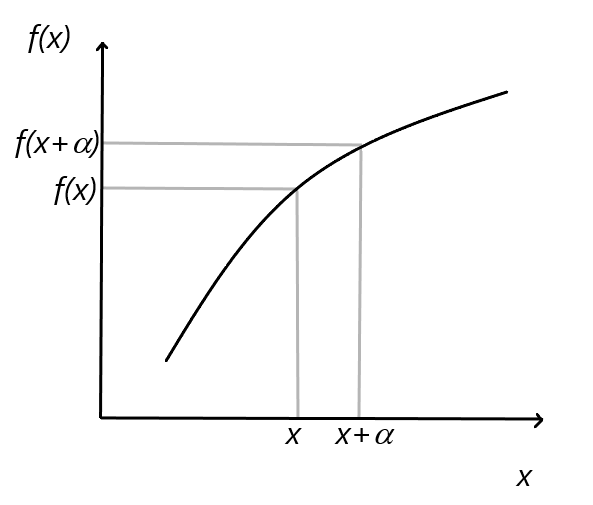
Limits are essential to Cauchy’s definition of continuity. Suppose we have a function f(x) such that, for every intermediate value of x between two given endpoints of an interval (on the x axis), f(x) constantly admits a unique and finite value. Suppose x is given an infinitely small increase α. The function f(x) is continuous in the variable x in the interval in question if the value of the difference f(x + α) − f(x) decreases indefinitely
as α decreases. Decrease indefinitely
means to become arbitrarily small as we decrease α. Thus it points to a process rather than a fixed entity. Yet Cauchy also refers to α as infinitely small,
thus eliding into treating an infinitesimal as though it were a fixed entity rather than a process.
Alternatively, one may say f(x) is continuous in x (in the given interval) if an infinitely small increase in x results in an infinitely small increase in f(x). This second definition is less precise, for we should also allow an increase of exactly zero for constant functions. If a finite positive increase in f(x) were possible no matter how long we indefinitely decreased the difference in x (for that is the only way to generate an infinitesimal quantity), then the function would effectively be discontinuous, jumping
in value at some x by some finite amount.
Since continuity is only definable on some finite interval and only by considering more than one value of x within that interval, one may define continuity in terms of the neighborhood
of x, which is an arbitrarily small interval surrounding some value of x. For a function to be continuous in x over some interval, it must be continuous in every neighborhood of some intermediate value of x, no matter how small we make that neighborhood. If a function should fail to be continuous in some neighborhood of a particular value of x, then it is said to be discontinuous at that value of x.
Continuity of a function implies a correlation between variations in the value of a function f(x) and the value of the variable x. By increasing x some small amount, f(x) likewise increases. If the difference in x becomes indefinitely small, so does the difference in f(x).
The epsilon-delta formalism of Karl Weierstrass (1815-1897) states more rigorously this notion that we can get arbitrarily close in values. To define the limit L of f(x) at x = c: For every ε > 0, there is a δ > 0 such that |x − c| < δ → |f(x) − L| < ε. A function f(x) is defined to be continuous on some interval of x if for all values of x within that interval, the following holds: For every ε > 0, there is a δ > 0 such that |x1 − x2| < δ → |f(x1) − f(x2)| < ε. The δ may be considered the radius of some neighborhood
of a value of x. For any small positive number ε, there will be some finite neighborhood of a value of x such that variations in the value of f(x) within that neighborhood will be bounded by ε.
The modern definition of limit requires there to be a single value, yet Cauchy’s definition allowed multiple values, so the function sin (1/x), though discontinuous at zero under modern definitions, because its value is not defined at zero, might be considered continuous under Cauchy’s definition. The increasing frequency of oscillation as x approaches zero implies that the slope of each successive wave approaches infinity or verticality, but never attains it. This notion of continuity is consistent with the intuition of being able to draw the graph of a function while always keeping the pencil on the paper and without ever having to lift it off the paper.
Modern mathematicians speak of functions as continuous, but historically natural philosophers were concerned with the continuity of geometric extension as such. To the extent that numbers can be represented geometrically, as points on a line, we raise the question as to whether and in what sense number is continuous. If it is admitted or stipulated that extension is continuous, the continuity of number may depend on whether we consider the number line as consisting of points and nothing more.
Ideally, we want to know whether there is anything missing
or gaps within a set of numbers represented as points on a line. In other words, we want to know whether it is possible in principle to define numbers with values intermediate to those in our set. Obviously, there are gaps between integers, which may be filled with fractions that are ratios of integers. The set of all ratios of integers is the rational numbers. By an unending process of division, we may generate rational numbers that are ever closer to each other, so that the distance between rational numbers approaches zero as the iteration of this process goes toward infinity. Does this mean that the rational numbers, which are thereby what we now call a dense
set, constitute a continuum or extension?
Georg Cantor (1845-1918) showed that the set of integers could be put in a one-to-one correspondence with the set of rationals, so that these two infinite sets are in some sense commensurate. He also proved that such a correspondence was impossible between the integers and the real numbers, and thereby defined that these were non-commensurate orders of infinity or cardinalities. He hypothesized, but did not prove, that there is no intermediate cardinality between that of the integers and that of the real numbers, the latter being known as the cardinality of the continuum.
What exactly is it about the reals that makes them a continuum,
and in what sense? Are the real numbers truly a continuum in the same sense as a geometric extension? Clearly density does not suffice, for the rational numbers are dense, but not a continuum. Although rational numbers can be situated arbitrarily close to each other, so that they might be regarded as continuous
in some sense, the absence of certain limits (points) within their quantitative ordering is problematic, for the rationals define a notion of magnitude for which they do not contain all possible values.
More concretely, when you have rational numbers, you have the operations of addition and multiplication, and their inverses, subtraction and division. The most generalized combination of these operations are algebraic formulas, yet we can construct algebraic equations for which no rational number is a solution. The simplest are of the form xn = p, where n is a positive integer and p is a prime number. We may approximate x by a series summation of rational numbers, but never come to it exactly. Indeed, it is not immediately obvious that x should be considered a number if we cannot express its value exactly, though we can always know whether it is greater or lesser than any given rational number. If we define algebraic numbers to be the solutions of polynomial equations with rational coefficients, or rather the roots of polynomial functions with rational coefficients, then there will necessarily be some algebraic numbers that are irrational or complex. The irrational algebraic numbers can at least be defined exactly with respect to the polynomial equation they solve, i.e., as a function of rational numbers with finitely many terms. Not all irrationals are algebraic. Indeed, Cantor showed that the algebraic numbers are countable, which means the vast majority of irrational numbers are non-algebraic or transcendental, and we do not arrive at the so-called infinity of the continuum even if we expand our notion of number to all algebraic numbers.
Moreover, Alan Turing proved that it is possible to create an algorithm for the decimal expansion of only countably many real numbers. The vast majority of irrational numbers do not have a calculable expansion, so we cannot say exactly where they are in the sequence defined by rational numbers. Even if we were to accept the equation of number with geometric point, we cannot know the exact location of most irrational points, not even as a limit. The location is knowable as a limit only for computable numbers, i.e., those real numbers for which a finite, terminating (though possibly recursive) algorithm can compute the nth digit in decimal, or equivalently, in any integer base. If being a number means having a definable value, it would seem that only computable numbers are truly numbers, and there are only countably many of these. Algebraic real numbers are a subset of the computable numbers. Other computable numbers include irrationals such as π and e, which are of utility in geometry and calculus, and appear in physical equations. Nonetheless, computable numbers in general have non-computable ordering.
It would seem that direct arithmetic or algebraic computation cannot bring us beyond countably many numbers to the infinity of the continuum. Nonetheless, Richard Dedekind famously constructed the real numbers out of the rationals by what he considered to be a purely arithmetic
approach, as it did not directly invoke geometric concepts, but instead worked solely from the rational numbers and the four arithmetic operations.[9] To achieve this, he first noted that inequality a > b can be defined in terms of subtraction, i.e., when a − b is positive. From such definition, we can show that (a > b AND b > c) → a > c, which is the same kind of ordering principle that allows us to compare the relative positions of points on a number line from left to right, without invoking geometry.
The rational numbers, we have noted, are dense in the sense that there are infinitely many rational numbers b between
any two rational numbers a and c, where between
can now be defined arithmetically as a > b AND b > c. We may divide the rational numbers into two classes (we do not say parts,
for that invokes geometry) A1 and A2, such that A1 is the set of all rational numbers a1 that are less than some rational number a, and A2 is the set of all rational numbers a2 that are greater than a, while a itself can be assigned to either class. This purely arithmetic separation into classes is analogous to cutting the number line in two pieces, one to the left and the other to the right of the point a. Dedekind now gives this condition for the continuity of a line, which cannot be proven, though it agrees with intuition:
If all points of the straight line fall into two classes such that every point of the first class lies to the left of every point of the second class, then there exists one and only one point which produces this division of all points into two classes, this severing of the straight line into two portions.
This definition seems to suppose that the line is constituted of points, but we need not assume that. Instead, let us consider all points on the line without asserting that the line is nothing more than a set of such points. Suppose there were more than one point, a and b that could sever a set of points A on the line into two portions, left and right, yielding the exact same subsets of points A1 and A2 whether we use a or b. Then we know there is some finite interval from a to b where there are no members of A. This would indicate greater discontinuity than even the rational numbers possess. On the other hand, suppose there was no member of A that could separate the two sets of points. That would mean either that no point at all could separate the two sets, or that such a point was not a member of A. Our supposition that every point of the first class lies to the left of the second class precludes the first option, unless we could have such a thing as adjacent points. That leaves the second option, that there is such a point on the line, but it is not a member of A, in which case A is not the full set of points on the line. Only in the case where A is the complete set of points on the line can we have exactly one member of A that separates the set into two portions as described. So Dedekind’s definition of continuity amounts to a definition of the set of all possible points on a number line, without necessarily identifying this set with the line.
Dedekind defines such a separation of the rational numbers into A1 and A2 as a cut (Schnitt). Every rational number defines exactly one such cut, once we pick a convention for including that number in the first or second subset. For such cuts, depending on choice of convention, either A1 has a greatest member and A2 has no least member, or else one A1 has no greatest member and A2 has a least member. Yet there can be cuts of the rationals that are not defined by any rational number. For such cuts, A1 has no greatest member and A2 has no least member. We can introduce a new, irrational number that is defined by that particular cut (A1, A2) of the rationals. Two numbers are considered different if and only if they make different cuts of the rationals.
Consider the (rational or irrational) numbers α and β corresponding to the cuts of rational numbers (A1, A2) and (B1, B2) respectively. If a and b are different, then the subsets A1 and B1 are not identical. If one of these subsets contained exactly one more member than the other, then both cuts would essentially be the same, differing only by the convention of whether the endpoint is included in the first or second subset, and a = b. So for differing a and b, it must be the case that there are at least two members of A1 that are not in B1, or vice versa. Supposing A1 to have the additional members a', a'' that are not in B1, there must be infinitely many rational numbers between a' and a'', which are also in A1 but not in B1. Thus α and β are definitely unequal. In the reverse case, where B1 has additional members b', b'' that are not in A1, there must be infinitely many rational numbers between b' and b'', which are also in B1 but not in A1. Since these are the only two cases in which α and β are unequal, we can define an ordering that includes irrational numbers, making α > β in the first case, and β > α in the second, and this ordering will be consistent with the ordering of the rationals. The ordering will also have the property that (α > β AND β > γ) → α > γ.
If the real numbers, the combined set of rational and irrational numbers, is defined as the set of all numbers that can make different cuts of the rational numbers, we find that the reals can be ordered on a line, subdivided into sets, and have infinitely many members between any two members of that set, much like the rationals. Yet the real numbers alone have a least upper bound
property, which we define as follows.
A subset S of F is defined to be bounded above if there exists some element b of F that is greater than all elements of S. Such an element b is called anupper boundof S.
A number b is called aleast upper boundof S if it is an upper bound of S and no number (in F) less than b is an upper bound of S.
Every non-empty subset S of F which is bounded above has a least upper bound that is an element of F, i.e., there exists some element b of F, such that b = sup S, wheresup Sdenotes the least upper bound or supremum of S.
This property is satisfied when F is the real numbers, but not by the rational numbers. For the rationals, we could define S to be the set of all rational numbers x s.t. |x2| < 2. That set has no supremum within the rational numbers, since there is no rational number such that |x2| = 2 and no smallest rational number in the set of those such that |x2| > 2.
Although the rational numbers are infinitely numerous and have a density such that infinitely many rationals lie between any two rationals, they lack the least upper bound property, which means there are definable quantities within the ordering of the rationals which are not themselves rationals. It is only when we augment the rationals with the set of all possible quantities that define cuts on the rationals, which is to say the set of all least upper bounds (and greatest lower bounds), that we arrive at a set that has the least upper bound property (and a greatest lower bound property).
Although Dedekind can only use computable irrational numbers as definite examples, the use of the principle of cuts on rationals to define the reals gives us a set with the least upper bound property, which makes integral calculus possible. More immediate consequences of the least upper bound property are: (1) the positive integers have no upper bound, since they have no least upper bound; (2) for every real number x, there exists a positive integer n > x; (3) if x > 0 and y is real, then there exists a positive integer n such that nx > y. This last implication, called the Archimedean property, is the arithmetic analogue of the statement that any line of length y, no matter how long, can be covered by some finite number of line segments of length x, no matter how short. This is another expression of our intuition of the continuity of the line, i.e., that we can compose the length from arbitrarily smaller lengths. Yet even the rational numbers have an Archimedean property.
The least upper bound property allows us to prove the intermediate value theorem, the analytic equivalent of drawing a graph without lifting the pencil from the paper, for the real numbers, which is not possible for the rationals.
Let the function f be continuous at each point of interval [a, b] (the set of all real numbers x such that a ≤ x ≤ b). Choose two points x1 < x2 in [a, b] such that f(x1) ≠ f(x2). Then f takes every (real) value between f(x1) and f(x2) somewhere in the open interval (x1, x2) (the set of all x s.t. x1 < x < x2).
At last we have a set of quantities, the real numbers, which has a degree of continuity that is analogous with our intuition of drawing a curve without interruption. These quantities are not in general calculable, however, though they can be placed in an ordering with the rational numbers, and indeed they are defined in terms of placements within this order. The arithmetic operations on rational numbers can be applied to the reals, retaining the same properties of closure, commutativity, associativity, etc. Yet the values of most real numbers are not computable by these operations. Can such non-computable numbers truly correspond to points on a line? We have seen that any quantity corresponding to a cut on the rationals must be a unique element in the ordering on rationals. If its value were spread over some finite segment, it could not define a unique cut of the rationals. So it would seem that we really can define continuity (of a graph) in terms of points. We have not proven that there can be no non-real quantities between reals; indeed one might define the surreals to augment the reals. But we do not need anything more than the reals to arrive at our intuitive notion of a linear continuum, and the reals can be defined arithmetically without direct reference to geometric concepts.
When the real numbers are represented in a coordinate geometry, they correspond to all possible endpoints of rays or line segments in a line. The length of any finite segment is given by the difference of the coordinate values of the endpoints: |b − a|. Thus the real numbers give us all possible lengths of extension, and these lengths are subject to the same arithmetic operations as rational numbers. By the infinity of the continuum, there are uncountably many possible endpoint positions and lengths, and the vast majority of these are not computable.
If we represent time as an extensive measure, we could say that instants are represented as uncountably many points, which constitute all possible endpoints of the rays that represent past and future. For finite line segments, the difference in coordinate values of the endpoints gives us the duration. Again, the vast majority of such values and durations are not computable. As movement requires duration, even the briefest movement traverses uncountably many instants. Instantaneous change, such as corruption, becomes more intelligible when we admit that there is a well-defined set of all instants after t
even though that set has no least member. The continuity of the reals does not abolish the logic of Burley’s rules of first and last instants. The least upper bound property guarantees that every subset of reals will have a least upper bound, but if the supremum of S is in S, then the complement of S will have no least member. If the supremum of S is not in S, then S will have no greatest member. Thus if we consider each instant t as making a cut (T1, T2) of the real number line representing time, and each of the two subsets represents a period of being or non-being, we arrive at Burley’s rules.
We could not arrive at such rules if we restricted our representation of time to the rationals, for then we could have scenarios where neither interval has a definite extremum (i.e., when the cut is made by an irrational number). Thus the continuity of time is necessary to establish Burley’s rules.
Since Burley’s rules of first and last instants are applied to successive beings, they should be applicable to movement, i.e., continuous change in place, quantity, or quality (or relations of these). Rest, unlike movement, is not a successive being, but a permanent being, something that remains over some period of time. It is senseless to speak of instantaneous rest. We can only know whether something is at rest by comparing its state over some interval between two instants. Knowing that something has velocity zero at some instant t does not tell us if it is resting, i.e., remaining in place for some time, or at an extremum, as in the case of a pendulum at one end of its swing. We regard a pendulum as being in continuous motion, and not at rest until it stops in an equilibrium position hanging straight down. Only when it has both zero acceleration and zero velocity do we consider something at rest. Such a state, once achieved, must persist unless the object is acted upon by some new force.
We saw earlier that a movement can have no first instant of being, whence it follows that the preceding non-being of movement does have a last instant. It need not follow, however, that any preceding rest should have a last instant, for rest is something more specific than the non-being of movement, as rest requires duration, whereas the non-being of movement might be found even in an instant. What of the other end of the movement, when the object comes to rest? Since rest requires some finite duration to elapse, there can be no first instant of rest, for if an object were already at rest in an instant, we could have instantaneous rest. Thus rest has no first instant, and movement has no last instant. This does not contradict Burley’s fourth rule, for we have defined rest more narrowly than the non-being of movement, thus allowing for an instant that contains neither rest nor movement. If rest were instead defined as simply the non-being of movement, then rest, per Burley’s rules, would need to have both a first and last instant, corresponding to the absence of a first and last instant of movement. As rest is defined in terms of the permanence of some physical state, it requires duration, and thus by the reasoning above, it can have no first instant, though we can say nothing about whether rest has a last instant.
If movement has no last instant, this does not imply that it is infinitely in the process of perishing, any more than its lack of a first moment implies that it is infinitely in the process of becoming. To avoid Zeno’s paradoxes, we must consider the division of movement as such (rather than division of the mobile object). Since time is the measure of movement, the divisibility of movement is the same as that of time, which is that of the continuum or cuts by real numbers.
We focus now on the transition from movement to rest, or coming to rest,
which may be considered a continuous (syneches) change. It need not be considered the mere decay or corruption of movement, but may also be construed positively, as coming to rest is the generation of rest. For clarity, we will use the example of local motion, but the same might apply to change in quantity (extension) and even alteration, insofar as things can become partially one quality or another, so they can undergo continuous change in quality.
We cannot speak of something coming to a stop, which is rest with respect to local motion, unless it is posited as currently in motion. If it is in motion, some time must elapse for it to do anything, since all motion requires some time. This principle was protested by Walter Chatton (c. 1290-1343), on the grounds that God might conceivably create a being instantaneously without time, so that it becomes
in an instant, but we are presently concerned with natural philosophy. If something is moving, how or when does it begin to be coming to rest
and at what point is it non-moving or at rest?
Zeno’s paradox of the flying arrow assumes that the continuum of time is composed of instants. Since the arrow in each instant is non-moving, for there can be no motion within an instant, it must be at rest, but two adjacent instants taken together would have the arrow in motion. We have seen that continuous magnitude is not properly composed of indivisibles, but rather these points act as limits, or in modern treatments, as possible cuts of the continuum. They are not parts of time, but the means of dividing time into parts. As both motion and rest require some duration of time, neither of them are to be found contained in any instant, though instants play an essential role in defining periods of motion and rest.
In modern terminology, something is coming to rest when it is decelerating, in the sense that the speed (magnitude of velocity) is decreasing. Deceleration requires the elapse of some time, so it has no first instant, though the initial limit of the period of deceleration may be defined by an instantaneous change from zero to negative acceleration, represented by a negative third derivative of position or jerk. If the deceleration terminates in rest, then there must be another change in acceleration, this time positive, bringing the object to zero acceleration in the state of rest. In idealized conditions, we might conceive of the acceleration instantaneously changing from some fixed negative number to zero, as when something with constant deceleration is brought to a complete stop by inelastic contact with some surface, so that upon attaining velocity zero, it does not reverse direction but comes to rest. This would indicate a discontinuity in the second derivative, acceleration, so that the third derivative or jerk is represented as a spike. In reality, there are no such discontinuities, but changes in acceleration may be sufficiently abrupt to be approximated as such. There is nothing in the nature of the continuity of time, space or motion that would prohibit a discontinuity in the higher derivatives of position, even if continuity of velocity is supposed (as in our example), so we may consider such an idealized scenario in the abstract.
The state of rest following the termination of deceleration has no first instant, so its non-being must have a last instant. It need not follow, however, that deceleration or coming to rest has a last instant, even on the assumption of discontinuity in acceleration. Indeed, the state of coming to rest is a type of movement, namely a continuous change in velocity. The continuity of velocity does not imply that the velocity function is differentiable; it may have a sharp bend, as when it decreases linearly but stays at zero. As a type of movement, coming to rest must have no last instant, and its subsequent non-being has a first instant. Thus there is an instant between coming to rest and rest that has neither movement nor rest. Although a thing is not moving within an instant that is within the duration of motion, it does not follow that it is at rest within that instant, for then anything in motion could always be considered at rest as Zeno says. One may admit the reality of an instantaneous state, without regarding it as either movement or rest.
In the above description, we have remarked that deceleration takes time, no less than motion. This is because acceleration can occur only when there is a change from one velocity to another velocity, and having any velocity requires the elapse of time. So-called instantaneous velocity is really a limit of velocity over arbitrarily small intervals. Similarly, the third and higher order derivatives all require some elapse of time. This does not result in a Zeno-type paradox of infinite regress preventing motion from getting underway, for the time required by infinite derivatives need not be additive, but are overlapping. Each velocity is defined between two points, and each acceleration is defined between two velocities, and so on, without paradox, due to the infinite divisibility of time.
Coming to a rest or stop has no first part, as can be proved by contradiction. If it did have a first part, there would be a primary time,
i.e., a finite duration that is the measure of that first part or beginning of coming to rest. That first part must consist of parts, for it were a unity there would be no movement within it, and we have seen that coming to rest must involve movement. Each of these parts would have a time that is a part of the primary time. The object must be coming to rest in each of these parts of the supposed primary time of coming to rest, for if it were to remain in a fixed state for any of these parts of time, it would be at rest during that time. Thus the first of these parts should instead be considered the putative primary time. The same argument can be applied to this new primary time and all subdivisions of its parts, so there is no first part of coming to rest.
When we speak of local motion and rest in the context of modern physics, it is understood that these are defined with respect to some given frame of reference. Since both motion and rest have no first or last instant, the relativity of motion and rest does not create any ambiguity or contradiction in this regard. There is an apparent contradiction, however, in making something a permanent being (rest) or successive being (motion) dependent on choice of reference frame, if this choice is merely conventional. The contradiction might be resolved if we regard inertial motion as a permanent state rather than a continuous succession of states, a definition that becomes intelligible if we deny the reality of absolute place, or at least hold that absolute position is irrelevant to the physical state of an object. If the physical state of an object is defined in terms of its ability to generate change in itself or in other objects, absolute position may be considered irrelevant to the physical state, as only relative velocity has kinematic effects.
For central forces such as the electric force, relative position or distance is also physically relevant, as a continuous change in linear position would have physical effects. Consider a frame where an electric charge moves linearly at constant velocity, stops abruptly (rapid deceleration) upon striking something inelastically, and remains at rest. While the charge is moving closer to some other charge, the two sources exert a stronger electric force upon each other, and the continuously varying strength of this force constitutes a successive change in physical state for both charged sources. The relativity of rest creates no paradox of simultaneous succession and permanence, for regardless of which rest frame we choose, the inelastic collision of the first charge with a barrier results in a cessation of the change of electric force strength. There would only be the change of state caused by any acceleration of the sources due to this force, if one or both of them are not held in place mechanically.
It is true that the choice of reference frame determines whether or not something is a moving charge, and therefore the presence or absence of magnetic force perpendicular to the direction of motion. Yet this will not cause the physical state of particles, in terms of magnitude of force experienced, to be static or changing depending on choice of reference frame. Rather a change of frame that nullifies
the magnetic force will instead result in the motion of a test charge being due to the electric force. The magnitude of total force may be different in each frame at relativistic speeds, but the static or changing nature of this magnitude will not depend on arbitrary change of reference frame.
When discussing coming to rest
with respect to local motion, we have retained the classical mechanical notion of rest meaning zero velocity and zero acceleration. In general relativity, however, the more relevant state of rest
in the sense of absence of violent motion would be freefall acceleration, which is never zero in a universe with other masses. Coming to rest
in this context would be the progressive negation of violent (non-gravitational) acceleration. If we define rest and local motion with respect to violent acceleration, we will once again have an absolute definition of rest and motion, or permanent and successive being.
Against Heraclitus’s assertion that everything is always in movement, so that there is only becoming or passing away but never being, Aristotle takes pains to show that all movement terminates. This is more easily evident in changes between contraries or other termini, as in qualitative and quantitative change between definite endpoints. He acknowledges, however, that local motion is not always between contraries, and since in principle it might continue indefinitely, the finitude of local motion must be qualified differently.
First, Aristotle argues against the possibility of traversing a finite distance in an infinite time. If a spatial interval AB is traversed over some infinite time cd, let us take some part of AB, call it AE, and suppose its length is 1/n times that of AB, where n is a positive integer. The time it takes to traverse AE must be less than the infinite cd, and therefore limited or finite. The same may be said of the other n − 1 intervals with the length of AE, so that the time to traverse AB must be a finite sum of finite times (which need not be equal since we do not assume uniform speed), and therefore finite, contradicting the hypothesis that cd is infinite.
This argument seems to falter on its supposition that a proper subset of an infinite extension must be finite, i.e., that any time less than an infinite duration must be finite. A modern mathematics student might casually retort that ∞/n = ∞ for any finite n, but this reply deals with quantity or measure without defining an arithmetic or geometric interval that is measured. In our problem, infinite time is not just a quantity, but the measure of some duration cd. The time c corresponds to a definite starting point, i.e., the time at which the mobile object is at A. If the length of cd is infinite, this means that the mobile object always approaches but never arrives at B. Thus there is no definite time d when the object reaches B, and cd really signifies a ray beginning at c and continuing indefinitely. It is easy to see that if we started the ray at some later point c', the length of the ray c'd would still be infinite even though it is a proper subset of the ray denoted cd. Yet any abridgement of the ray from the right side rather than the left would make a finite line segment, assuming that the new endpoint d' must be definite, as it must if the intermediate spatial point E is definitely traversed.
The ray cd contains the set of points representing times at which the mobile object (or its center, its edge, or some other definite point of it) occupies some point on the spatial interval AB. Similarly, cd' contains the points of time at which the mobile occupies points in AE. If cd' could be infinite, then the mobile always approaches but never arrives at E. This means it never traverses any part of EB. Yet this contradicts our initial supposition of movement across the entire interval AB.
Taking another approach, if we accept that a mobile object has some definite speed over each finite segment of distance, it follows that it is impossible to move a finite distance in infinite time. For each finite segment of distance traversed with some definite speed, it would take some finite amount of time to travel. Any finite interval AB is composed of finitely many finite segments, however small they may be, and a finite extension cannot be composed of infinitely many finite extensions, so the total time must be finite, however much the speed, though finite, may vary from one arbitrarily small but finite subinterval to another. There are but two ways something could move a finite distance in infinite time. First, the velocity could be zero for some infinite period of time, but in that case we are no long speaking of a single movement, for it is interrupted by a period of rest. Moreover, if the velocity becomes zero before reaching B, then the subsequent infinity of time at velocity zero means that the movement from A to B is never completed. If the velocity becomes zero upon or after reaching B, then the subsequently infinite time would be a period of rest that is superfluous to the movement from A to B, which was already completed in a finite time. The second way is to posit that the velocity is infinitesimal when traversing some finite spatial interval of length s ≤ AB. The mean velocity while traversing s may be represented as s/∞, the ratio of distance to time. This ratio has no definite value, as it is indistinguishable from r/∞ for any finite value of r. Thus the mean velocity would have no definite value, and we must deny any definite physical reality to velocity and any physical property dependent on it. Such a supposition is inconsistent with the notion of movement as a continuously occurrent change.
Likewise, the mobile cannot travel an infinite distance in a finite time. For each segment of duration, the body must have moved some finite distance (or else it is at rest). If the total time is finite, it can only be composed of finitely many such segments, so the total distance traversed must likewise be finite. We cannot have infinite velocity, since velocity is a ratio, and infinity
as the ratio 1/0 is not some large quantity, but a quantitative indefiniteness. It is something approached, never reached. Infinite
speed would require some jumps or discontinuities across space, which is contrary to what we mean by movement, a continuous change between termini.
The above argument follows from the definitional requirement that movement is continuous, and holds for local motion only insofar as we require that to be continuous or non-saltational. Quantum mechanics apparently allows jumps
across space, which would be exempt from this argument, as we are no longer dealing exclusively with movement through space. If these jumps really do occur, they must be accounted for by the continuous evolution, i.e., movement, of something extra-spatial, e.g., a wavefunction or field in a more abstract phase space. It is unclear, however, if such jumps ought to be regarded as really occurrent, or if they are truly examples of infinite speed. For one thing, we can only have imperfect knowledge, even in principle, of the positional state prior to observation, and for another, we never make successive observations of a particle at locations and times requiring infinite or even superphotonic speed. Insofar as the time-dependent wavefunction is regarded as the continuing evolution of a propensity or potentiality to realize various possible states, we may question whether this wavefunction, being mere potentiality, ought to be subject to any restrictions against infinitely quick or abrupt changes. Here the restriction seems to arise solely from the mathematical form of the wavefunction, insofar as we require it to be a continuous function. Yet this mathematical requirement of continuity follows from the physical requirement of finite momentum.
A relativistic conception of velocity does not alter any of these arguments, since they all suppose we have chosen a particular reference frame for defining velocity, and the same problems will arise no matter which frame we choose.
Similar arguments may be applied to show that there cannot be an infinite movement, i.e., a movement with infinite magnitude between termini, in a finite time. This applies to local motion no less than other kinds of movement. Any finite time can be divided into finitely many finite parts. In the first such part, only a finite extension (of space, qualitative degree, or quantitative increase) can have been traversed. The same holds for all other parts of time, and since there are finitely many of these, the sum of the extension traversed in that time must be finite. Moreover, to traverse an infinite magnitude in a finite time would require infinite velocity, with the problematic indefiniteness which that would entail, and would be contradictory with the continuity of movement.
If we conceive of the mobile object as corporeal and finite in extension, we may ask whether this finite object can traverse an infinite magnitude in a finite time. The argument against infinite movement in a finite time may be applied here. The finite time may be divided into finitely many finite parts. During any one of these parts, the finite object can move only a finite distance (again, assuming speed must be finite), and thus the sum of the distances traveled in a finite time must be finite, even if speed is not uniform.
Suppose we had a divergent speed, such that it doubles after a half second, doubles again after a quarter second, and doubles again after an eighth second, etc. If it travels one spatial unit after the first half-second, then its total distance traveled would be the infinite sum:
∑ 2n for 0 ≤ n ≤ ∞
This infinite movement would occur over a finite time, for the limit of t as n → ∞ is 1. Yet we seemingly have not violated the requirement that only a finite distance is traversed in every finite interval. That non-violation is only apparent, however. For, by making the time intervals ever smaller and thus creating an infinite series, we never truly complete the finite time interval, i.e., we never arrive at t = 1. This is just another Zeno-style paradox. If we confine ourselves to physical reality, it is unacceptable to have t = 1 impossible to reach. We must therefore have some interval of time in which an infinite distance is traversed, in which case there is a discontinuity. Interpreting the asymptotic function physically, the discontinuity is at t = 1, for the mobile is nowhere
when t = 1.
Since a mobile object of finite magnitude cannot traverse an infinite space in a finite time, it follows conversely that an infinite magnitude (if it could exist) cannot traverse a finite magnitude in a finite time. For it does not matter whether we think of the finite as the thing that is moving across an infinite space or suppose that the infinite is the thing moving across something finite, as both would take the same amount of time, and it is just a question of which thing we regard as fixed, i.e., our choice of Galilean reference frame. For the infinite A to traverse the finite B, first one finite part of A must traverse B, then another part of A, and so on indefinitely. This would be the same amount of time as for the finite B to traverse each of the parts of A, and thus the time for the infinite to traverse the finite is the same as for the finite to traverse the infinite. Since the finite cannot traverse the infinite in a finite time, neither can the infinite traverse the finite in a finite time.
All of these arguments suppose that the spatial interval under discussion is to be traversed but once, not repetitively. Naturally, if the same finite interval could be traversed repeatedly in a single motion, then in that sense the finite might be traversed in an infinite time. If we are dealing with linear motion, however, this would involve reversals of directions, each of which might be considered to be a break in movement, as in reflection. With simple harmonic motion, however, we can have such reversals in a single movement that may continue for many periods of oscillation. While the period remains constant for as long as the harmonic motion persists, even the most minuscule energy loss of the system will cause harmonic oscillation to dampen, i.e., decrease in amplitude, and eventually terminate. In physical reality, the only single movement that can be infinite in time, under modern mechanics no less than under Aristotle’s paradigm, is rotatory local motion, as Aquinas remarks. [Comm. on Physics, 842; Physics VIII]
The allowance of at least one type of simple motion that can be infinite in time may seem to undermine Aristotle’s endeavor to refute Heraclitus. The doctrine of Heraclitus, however, is objectionable only insofar as it would make everything becoming or passing away without ever being anything, as if movement never resulted in the realization of any physical state or definite form of the mobile. If this were so, we would have the paradoxical if not incoherent situation of having a world full of movement without any subjects of movement. A body that rotates without any limit in time does not generate such paradox. First, its rotational motion, though infinite in time, is not infinite in nature, for it is fully comprehended in a single finite rotation, which may repeat indefinitely. We can fully describe its dynamical state in terms of fixed concepts, having a certain center of rotation and angular velocity, neither of which change. Linear motion may also continue indefinitely, but only if the infinity of time is matched by an infinity of space. That is to say, the space traversed must increase indefinitely with time, though it is finite at any given point in time, if time has a beginning. If time has no beginning, it would still be problematic to posit linear motion without beginning, since that would require an actual infinity of space.
What has been said about motion with respect to finite and infinite magnitudes applies in particular to coming-to-rest, which is a kind of movement. Thus all becoming or passing away must be finite in time insofar as it is finite in the magnitude traversed between termini, be they qualities, quantities or places (or relations between these). The exception of rotational motion does not contradict this, for it repeats the same states over and over again, and the becoming and passing away of various angular states is grounded in the fixed being of the rotational state of the body, defined by its center of rotation and angular velocity. In modern mechanics, conservation of angular momentum is absolute and universal, so that we would consider such perpetual movement to be a mark of stability rather than flux.
In most Greek cosmologies, circular motion was the model of eternity, as it was believed that the heavens revolved around the earth forever. We instead recognize rotation about an axis as the only infinite motion, but for the purpose of illustration we might consider the ancient heavens as rotating concentric spheres, as in the model of Anaximander which was incorporated by Ptolemy and long upheld by European astronomers. These eternal rotations should be kept in mind when interpreting the prime mover argument. With this understood, there should be no mistaking priority of movement for priority in time, for all such heavenly motions were supposed to be contemporaneous and eternal. This ever-moving cosmos was not considered to be in unending flux, but was a model of stability that mirrored the Greek philosophers’ notion of divinity: serene, unperturbed, and everlasting.
Continue to Part VIII (under development)
[1] See discussion in: Ian J. Thompson. Philosophy of Nature and Quantum Reality (2010), 6.2
[2] Ian J. Thompson. Real Dispositions in the Physical World.
British Journal for the Philosophy of Science, v.39 (1988), pp. 67-79.
[3] The point-like
particles of modern physics are not merely geometric points, or indeed they would be incapable of interaction in space, occupying no finite part of space. Rather, they can be considered centers of force or fields which have various radii of interaction, depending on the type of interaction. They are point-like
only in that their radius of impenetrability is effectively zero (or at any rate smaller than can be measured).
[4] Edith Dudley Sylla. Mathematics and Physics of First and Last Instants: Walter Burley and William of Ockham,
in: Frederic Goubier and Magali Roques, eds. The Instant of Change in Medieval Philosophy and Beyond (Brill: Leiden, 2018), p.103.
[5] Cecilia Trifogli. Walter Burley on the Incipit and Desinit of an Instant of Time,
in:
Frederic Goubier and Magali Roques, eds. The Instant of Change in Medieval Philosophy and Beyond (Brill: Leiden, 2018), p.85.
[6] Sylla, op. cit., p.107.
[7] There may be a neurological basis for the number line’s intuitive appeal. Human infants and other animals have been observed to spontaneously associate lower number with space on the left and higher number with space on the right. Rugani et al. Animal Cognition. Number-space mapping in the newborn chick resembles humans’ mental number line.
Science. 2015 Jan 30; 347(6221):534-6. doi:10.1126/science.aaa1379.
[8]
David Tall, Mikhail G. Katz. A cognitive analysis of Cauchy’s conceptions of function, continuity, limit and infinitesimal, with implications for teaching the calculus.
(2014) https://arxiv.org/abs/1401.1468
[9] Richard Dedekind. Essays on the Theory of Numbers. Translated by Wooster Woodruff Beman (Open Court Publishing: Chicago, 1901), pp.3-27.
© 2022 Daniel J. Castellano. All rights reserved. http://www.arcaneknowledge.org
| Home | Top |